Golden section – geometric construction
The Golden Ratio is found in nature, art and architecture.
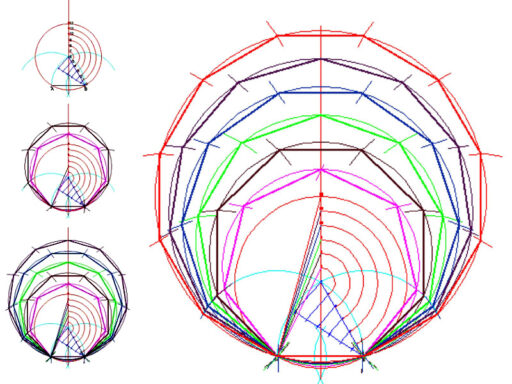
Graphic construction of the golden section
The Golden Ratio, also known as the “golden proportion” or “divine proportion”, is a special proportion found in nature, art and architecture. It is a mathematical relationship that occurs when the ratio of the sum of two quantities to the larger quantity is equal to the ratio of the larger to the smaller quantity.
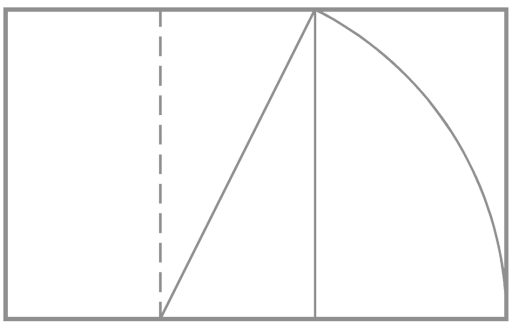
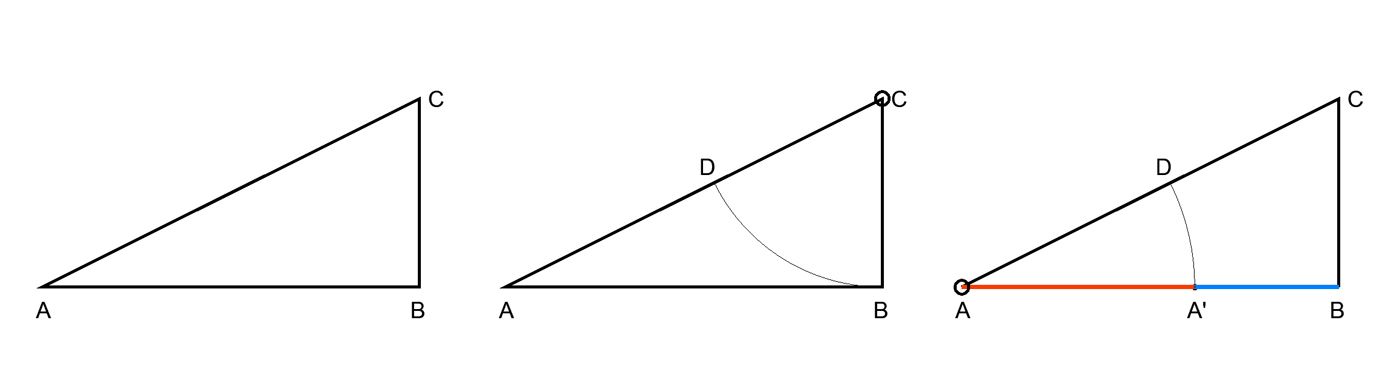
Geometrically, the Golden Ratio can be constructed as follows:
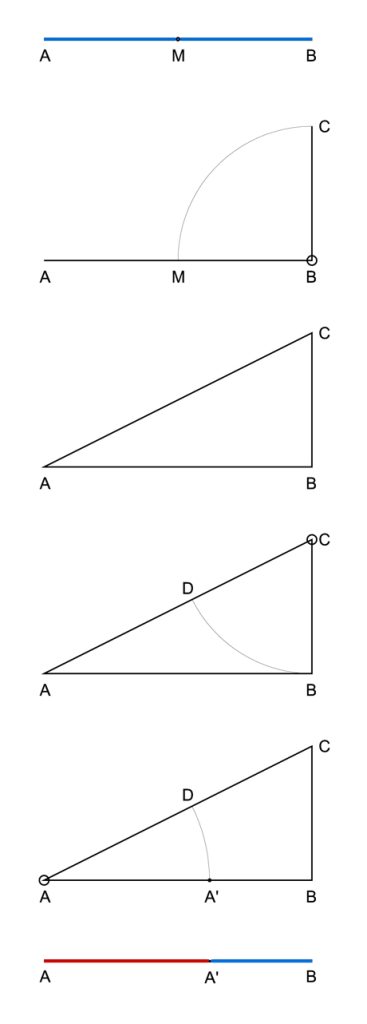
Draw a segment A-B
Conduct the perpendicular from an end (B) of length corresponding to half of the segment A-B
Join the two ends of the segment to obtain a right triangle
With a compass, center on C and draw an arc with radius C-B until it meets the hypotenuse A-C obtaining point D
With the compass, center at A with distance A-D and make an arc until you meet the segment A-B obtaining point A’
The distance A-A’ represents the golden ratio of the segment A-B
This split point, A’ has some interesting properties.
For example, if the segment AB is considered as the base of a rectangle and a square is drawn on the smaller side of the rectangle, the ratio of the length of the smaller segment to the length of the larger segment will be approximately equal to the Golden Ratio.
This property has been used extensively in architecture and art to create aesthetically pleasing and harmonious proportions.