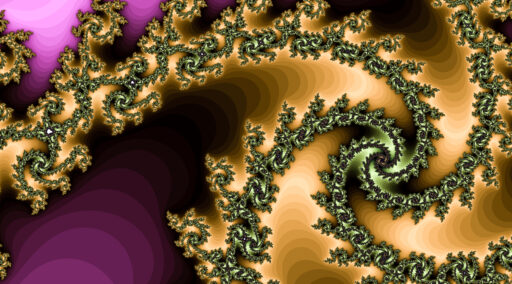
Fractals: the geometry of nature

They are as fascinating as a landscape and more precise than a perfect drawing

by Antonio Cianci and Alessandra Di Lorenzo

One of the main activities of the human intellect is to try to represent nature. Artists and scientists have tried over the centuries, with the tools of their disciplines, to provide a view of the world that is as close as possible to their perception. Over the centuries, however, art and science have become increasingly detached in their respective depictions of the world. For a long time, artists concentrated on the most beautiful and most common elements of the world around them such as panoramas, portraits, still lifes. Scientists, at the opposite extreme, defined abstract models that simplified nature in order to be able to describe it with a few and, as far as possible, simple rules. With the 20th century, this trend was reversed. New art forms approached geometry, new mathematical theories and new calculation tools made it possible to give a visual representation to complex mathematical models and theories, moving from the geometry of the mind to the geometry of nature. But let us try to understand how this may have happened.

The geometry of the mind

For most of this century, the terms mathematics and science have designated disciplines too distant and complex for the general public to understand and appreciate. For most people, mathematics is a dry discipline, devoid of any contact with the real world. This perception stems from the time of Euclid of Alexandria, the Greek mathematician who, around 300 BC, formalised geometry as we know it and whose ideas have dominated Western thought ever since. Starting from intuitive axioms, such as a line possesses infinite length, Euclid developed a coherent set of logical rules to describe points, lines and simple shapes. However, his geometry was an abstract universe with no connection to everyday reality: he started from intuition and derived his entire theory by applying pure logic to his axioms. At the time, in fact, no man of culture would have allowed his intellect to compromise with reality and therefore observation never played a relevant part in Euclidean theories.

“Science and art… we have become accustomed to seeing them as opposite poles, but do they not depend on each other? Is not even the thinker, who seeks to understand natural phenomena in an attempt to reduce complexity to a few fundamental laws, the dreamer who immerses himself in the richness of forms and sees himself as part of the eternal play of natural events?”

H.O. Peietgen, The beauty of fractals, 1986

The geometry of science

It was not until the early 1600s that the French philosopher Descartes brought Euclid back down to earth. To measure space he subdivided it using three mutually intersecting perpendicular lines (the Cartesian axes), allowing any existing object to be assigned a precise position. In this way, the entire universe could be described as an ordered whole. This view is now the basis of modern science. The idea of whole dimensions is now so ingrained that one hardly realises how revolutionary it is. Indeed, prior to his theories, space was perceived in terms of objects and events, rather than abstract measurements. Descartes’ vision was completed the following century by Isaac Newton and Baron Gottfried von Leibniz who, separately, defined the principles of differential calculus. Newton and Leibniz’s fundamental idea was to transform curves into straight lines. Leibnitz in particular stated that all curves consist of infinitely small segments (called tangent or derivative lines).
As a curve becomes larger, it becomes more and more similar to a straight line. Differential calculus provides the tools to identify these straight lines, the tangent lines, which are the core of almost all modern science and mathematics. Nowadays, everyone from architects to economists use differential calculus (and its inverse, integration) to formulate the system by which the known universe is described. The importance of infinitesimal calculus is enormous. Starting with the theory of gravitation, completed by Newton right after the definition of these concepts, passing through physics and economics, everything is based on the assumption that every curve consists of an infinite number of segments. There were, however, some obscure points, and from them one of the most important mathematical theories of the 20th century, fractal geometry, would develop. But let us proceed in order.

The crisis of classical mathematics

In the 19th century, most mathematicians were still bound to the studies carried out centuries earlier by Euclid and the theories of Newton and Leibniz and were convinced that there was nothing more to discover. They were proud of their mastery over everything that was strange and irregular; they even went so far as to refer to curved lines as bent straight lines. It is well known, however, that the certainties of one epoch represent the problems of the next. As early as 1875, the German mathematician Carl Weierstrass described a curve with decidedly strange characteristics, even considered pathological and unpleasant by his colleagues as they questioned the concepts of distance, area, space and dimension. Others, such as the German Georg Cantor and the Pole Waclaw Sierpinski, obtained lines and geometric figures whose length and area could not be calculated.

Fractals are more than just a mathematical curiosity: they offer a concise method for describing objects and formations. Many structures have a geometric regularity, called invariance with respect to scale change or self-similarity. If one examines these objects at different scales, one always encounters the same basic elements.

In 1890, even the Italian Giuseppe Peano proved that a continuous curve with no surface can fill a region of space. The mathematicians of the time, not really understanding the answer, hoped that the problem would never happen again. Obviously it did not. As time went on, the new, pathological and unpleasant shapes came to create a veritable ‘gallery of monsters’. Indeed, scholars, accustomed to the study of simpler forms, had to get used to a new structure of the universe and, therefore, to a new conception of surface and dimension and, above all, they had to agree that it was precisely the ‘pathological forms’ that were revealing themselves day by day to be more and more inherent to the surrounding reality. Meanwhile, Felix Hausdorff and Abram S. Besicovitch even redefined the concept of dimension. They added a fourth and fifth dimension, even higher integer dimensions and, above all, fractional dimensions, such as 1.5 or 2.3, which could be calculated by means of special algorithms. But it would be more than fifty years before anyone was able to answer the questions raised in the early years of the century.

The geometry of nature

In 1958, the French mathematician Benoit Mandelbrot was hired at Ibm’s Tomas J. Watson research centre to work on a project investigating systems for eliminating noise that disturbed digital transmissions. When he examined this noise, Mandelbrot discovered that it had an intricate structure by which the technology being developed would not be able to keep the problem under control. He realised that it was simply impossible to control or predict it. It was in fact chaos. However, the speed with which Mandelbrot responded to the noise problem did not stem from his profound knowledge of telecommunications technology, which he did not know, by the way. He succeeded because this problem had remarkable similarities with the price of cotton. Since the early 1950s, Mandelbrot had devoted himself to the study of the prices of consumer goods, in particular that of cotton, on which reliable data from centuries of trade was available. In his studies he observed that the cost of cotton behaves with a strange kind of recursiveness: its variations are very similar whether they are referred to years, months or decades.

In practice, if you zoom in on a graph of cotton price trends over time, each part has roughly the same trend as the whole. Mandelbrot called this similarity invariance of scale. Many other unpredictable phenomena such as river floods or stock market trends show the same cyclical structure. However, the word cyclic should be understood in a different sense, not in the classical sense of the term. In Mandelbrot’s definition they consist of cycles that contain others and so on ad infinitum, even though none of the cycles repeat exactly. After noting this behaviour, Mandelbrot also began to understand it from a mathematical point of view, but he could not convince anyone of the validity of his theories. His equations were too abstract and his conclusions too inconvenient. In the optimistic 1950s, who could wish for a theory that claimed things were complex, uncontrollable and chaotic? So it was that for over a decade Mandelbrot’s bizarre ideas remained only his personal obsession.

From a technical point of view, a figure is not a fractal if its detail is not infinite: in a fractal, each of its parts resembles the whole, whatever the scale at which it is displayed.

In 1968, however, things changed. Mandelbrot had begun to study the recurring patterns in the Nile’s level fluctuations, also mentioned in the Bible. Together with a hydrologist, he surveyed all the levels and, having completed his studies, put them together in a series of graphs. In addition, using his formulas he produced other graphs, fakes, that showed similar behaviour to the real graphs. When he showed them to a group of eminent hydrologists, no one was able to distinguish the real graphs from the fake ones, while the graphs that had been prepared based on traditional calculation models were immediately recognised as fake. The realism of Mandelbrot’s graphs showed that he was approaching something truly powerful in describing nature as it really is and not as it is represented in the minds of mathematicians. Comforted by these successes, he continued to produce fake graphs depicting other chaotic phenomena: stock market and commodity prices, mountains and coastlines. In all his experiments, the visual characteristics of his images were fascinating. As he himself said, ‘first people escaped my writings, then no one could escape my graphs‘.

Anticipating a concept that would become fundamental in modern fractal geometry, Johann W. Goethe wrote that in order to progress into infinity one must explore the finite in all dimensions.

The geometry of art

But his work did not end there. Once he had explored self-similar fractals with which to describe nature, Mandelbrot defined a number of iterative procedures that were used to produce abstract mathematical constructions, such as the famous sets that took his name. Finally, in 1982 he published the seminal The Fractal Geometry of Nature coining the term fractal (from the Latin frangere, meaning to break up into irregular fragments), completing the work that Weierstrass, Cantor, Sierpinski and others had begun at the turn of the century.

Even before they were recognised mathematically, the images produced through Mandelbrot’s algorithms became incredibly famous. Mathematical artists such as Richard Voss, Greg Turk and Alan Norton slightly modified Mandelbrot’s basic procedures to create stunning panoramas, both realistic and abstract. This rediscovered link between art and mathematics gave new life to the latter. The modern theories of relativity and quantum mechanics, however beautiful and fascinating, in fact require years of study before they can be understood. With fractal geometry, however, thanks to computers, even the layman is able to appreciate the most abstract fractal image.

All figures are fractals

In the early 1980s, mathematician Michael Barnsley joined the group of those studying fractals. Passionate since childhood about ferns, he observed how each frond resembled the whole plant and wrote a simple computer programme that modelled this feature. The resulting image was far more realistic than he expected and quickly became one of the most famous fractals. More importantly, in 1985 mathematician John Elton (no relation to the more famous singer) proved that any image can be represented by a class of fractals.

This was a huge step forward for a community that was immersed in these figures without any logical connection between them. The first important application of their work was image compression.

Barnsley had in fact succeeded in compressing complex images into very small codes by translating them into fractals and achieving compression ratios of over 10,000 to one in some cases. Fractal compression of images implies rather interesting new possibilities such as sending video animations in real time using ordinary telephone lines. Lately, fractals have become increasingly popular: they are used in film productions for special effects, computer graphics rendering systems use them to create natural textures, and scientists and mathematicians make them a fundamental tool in their studies. Science and mathematics are no longer seen as the barren, charmless terrain of previous centuries.

Source: Mondadori Informatica S.p.A.